Blog

Parin Sir > Quantitative Aptitude (Maths) > Circle > Some Important Results > Some Important Results

Some Important Results

August 8, 2020
Category: Some Important Results

☼ Some Important Results :

The perpendicular drawn through the centre of a circle on a chord bisects the chord.

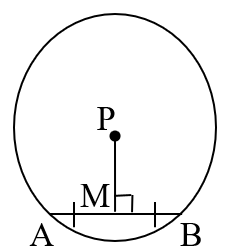
$\large \mapsto$ In the adjoining figure, $\overline{AB}$ is a chord of a circle ⊙ ( P, r ) and $\overline{PM} \bot \overline{AB}$ , then $\overline{PM}$ bisects $\overline{AB}$ at M.

i.e. M is the mid–point of $\overline{AB}$ .

Some important results :

The perpendicular bisector of a chord of a circle, lying in the plane of the circle, passes through the centre of the circle.
Three distinct collinear points cannot be the points on the same circle.
One and only one circle passes through three distinct non–collinear points.
If two chords of a circle bisect each other, both of them are diameters of the circle.
In the same circle, if two chords other than a diameter are given, the distance of the longer chord from the centre is less than the distance of the smaller chord from the centre.
If two distinct points are on two circles, then it is said that both the circles intersect and those points are called their points of intersection.
Two distinct circles cannot intersect each other in more than two distinct points.
If one and only one point is on two circles, then it is said that both the circles touches and that point is called their point of tangent.
In the same circle ( or in congruent circles ) congruent chords are equidistant from the centre ( centres ) of the circle ( circles ).
If $\overline{AB}$ and $\overline{AC}$ are congruent chords of ⊙ ( P, r ), then AP bisects $\angle$ BAC.

Common chord : If two distinct circles intersect each other in distinct points A and B, then $\overline{AB}$ is called the common chord of the two circles.