Blog

Parin Sir > Quantitative Aptitude (Maths) > Co–Ordinate Geometry > Lines > Concurrent lines > Concurrent lines

Concurrent lines

No Comments

☼ Concurrent lines :-    If three or more distinct coplanar lines pass through a single common point, they are said to be concurrent lines and that point is called their point of concurrence.

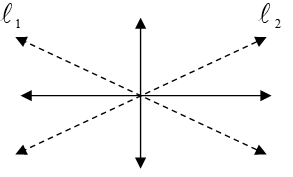
e.g. In the adjacent diagram  lines  l1,   l2, and  l3 are called  concurrent lines   and   the   point   P  is called  the point of concurrence.

\mapsto  The  necessary and sufficient conditions for three distinct lines

l1  :  a1x   +  b1y  +  c1  =  0          :  Where,  a_1^2 + b_1^2 \neq 0

l2  :  a2x  +  b2y  +  c2  =  0         :  Where,  a_2^2 + b_2^2 \neq 0

l3  :  a3x  +  b3y  +  c3  =  0         :  Where,  a_3^2 + b_3^2 \neq 0

to be concurrent are :

 (i) a1b2 – a2b1 \neq 0, (ii) a2b3 – a3b2 \neq 0, (iii) a3b1 – a1b3 \neq  0,

and     (iv)   \left|\begin{matrix}a_1&b_1&c_1\\a_3&b_2&c_2\\a_2&b_3&c_3\\\end{matrix}\right|= 0

☼ The equation of  a line passing through the intersection of  l1 : a1x  +  b1y + c1 = 0   and   l2 :  a2x  +  b2y  +  c2  =  0  is :

a1x  +  b1y + c1  +  \lambda (a2x  +  b2y  +  c2)  =  0  :  \lambda \in R

☼ The equations of  angle bisectors of  two intersecting lines l1 : a1x  +  b1y + c1 = 0   and   l2 :  a2x  +  b2y  +  c2  =  0   are :

\frac{\left|a_1\mathrm{x\ } +\mathrm{\ } \mathrm{b}_1\mathrm{y\ } +\mathrm{\ } \mathrm{c}_1\right|}{\sqrt{a_1^2\ +\mathrm{\ } \mathrm{b}_1^2}}   =  \pm \frac{\left|a_2\mathrm{x\ } +\mathrm{\ } \mathrm{b}_2\mathrm{y\ } +\mathrm{\ } \mathrm{c}_2\right|}{\sqrt{a_2^2\ +\mathrm{\ } \mathrm{b}_2^2}}

Click here to see next topic

Share
educational sutra maths sutr Examples mathematics SUMS ganit example concept math proof Parin theorem sir theorems bhai definition Mehta definitions maheta fundamental classes basic class base education sum online dakhla tutorial formula

Leave a Reply